【Read between lines】Peskin, Schroeder "An Introduction to Quantum Field Theory" p. 301 (derivative of determinant in Penrose graphical notation for Gaussian integral of Grassmann numbers)
Key Words
Grassmann number
Gaussian integral
Determinant
Levi-Civita symbol
Penrose graphical notation
Relevant section
Similarly, you can show that
$$
\left(\prod_i\int d\theta_i^\ast d\theta_i\right)\theta_k\theta_l^\ast e^{-\theta_i^\ast B_{ij}\theta_j}=(\det B)(B^{-1})_{kl}.
$$
Inserting another pair $${\theta_m\theta_n^\ast}$$ in the integrand would yield a second factor $${(B^{-1})_{mn}}$$, and a second term in which the indices $${l}$$ and $${n}$$ are interchanged (the sum of all possible pairings).
Solution
First, in this article you need knowledge about Penrose graphical notation.
We can show the relations via derivatives:
$$
\left(\prod_i\int d\theta_i^\ast d\theta_i\right)\theta_k\theta_l^\ast e^{-\theta_i^\ast B_{ij}\theta_j}=\frac{∂}{∂B_{kl}}\left(\prod_i\int d\theta_i^\ast d\theta_i\right)e^{-\theta_i^\ast B_{ij}\theta_j}=\frac{∂}{∂B_{kl}}(\det B).
$$
Determinant is represented as
$$
\det B=\frac{1}{n!}\epsilon_{1\cdots n}^{i_1\cdots i_n}\epsilon_{j_1\cdots j_n}^{1\cdots n}B_{i_1}^{j_1}\cdots B_{i_n}^{j_n}.
$$
This is shown in the following notation:
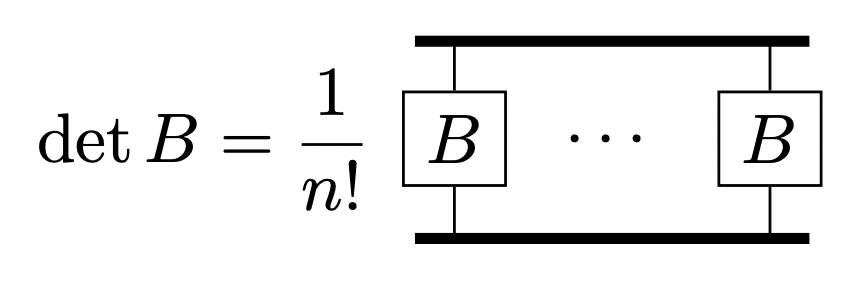
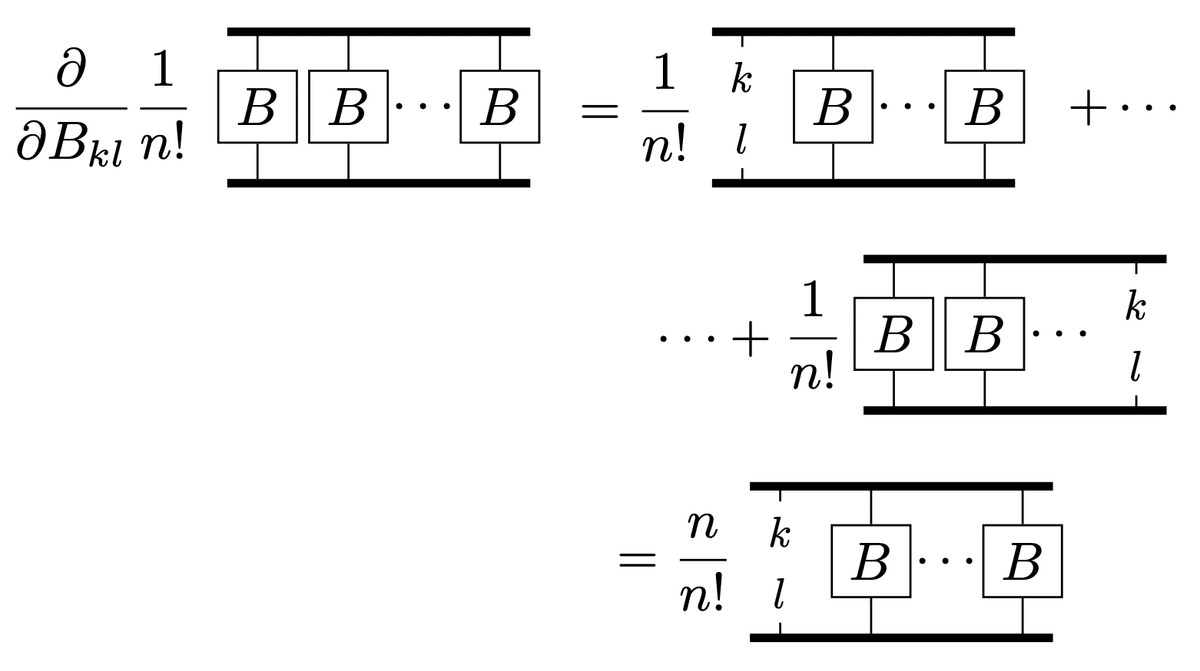
The derivative by $${B_{kl}}$$ is,
On the other hand, $${B^{-1}}$$ is
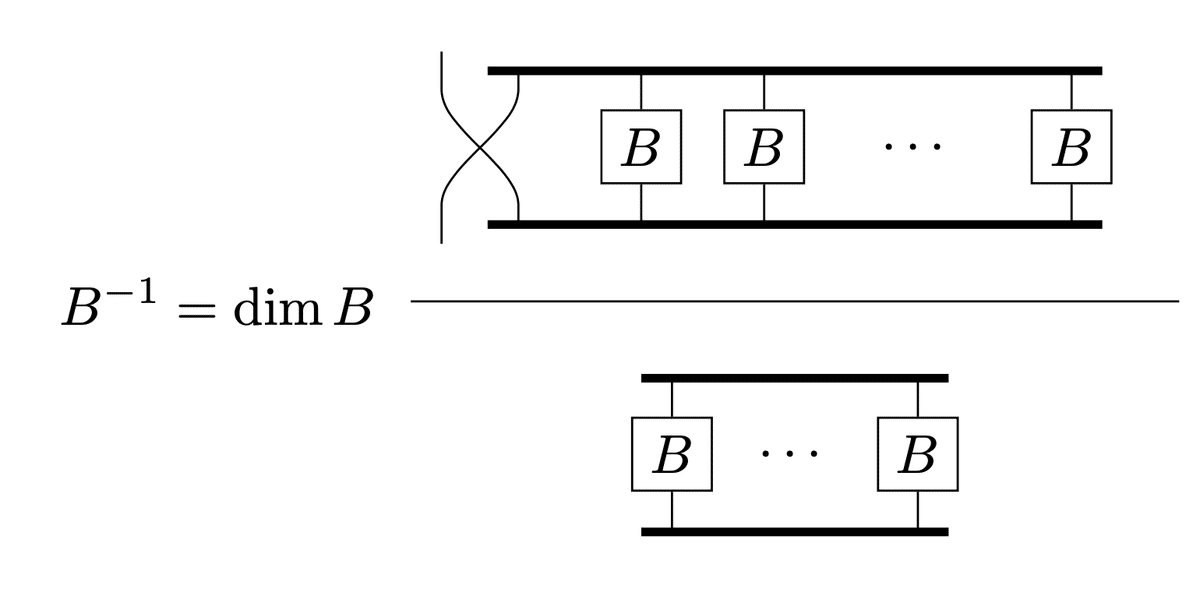
so $${∂\det B/∂B_{kl}=(B^{-1}\det B)_{kl}}$$.
この記事が気に入ったらサポートをしてみませんか?
